There, where worlds seem, with slow steps,
Like an immense and well-behaved herd,
To calmly graze on the ether’s flower.
Giovanni Pascoli, Il Ciocco

A question often asked by the general public interested in cosmology about the expansion of the Universe is the distance scales on which it effectively acts. Before commenting on this, let me recall first some historical facts.

In 1927, Georges Lemaître published a revolutionary article in the Annales de la Société scientifique de Bruxelles entitled “Un univers homogène de masse constante et de rayon croissant, rendant compte de la vitesse radiale des nébuleuses extragalactiques” (“A homogeneous universe of constant mass and increasing radius, accounting for the radial velocity of extragalactic nebulae.” As the title suggests, Lemaître showed that a relativistic cosmological model of finite volume, in which the Universe is in perpetual expansion, naturally explains the redshifts of galaxies, which at that point were not understood. In particular, the article contained a paragraph establishing that forty-two nearby galaxies, whose spectral shifts had been measured, were moving away at speeds proportional to their distances.

Lemaître gave the numerical value of this proportionality factor: 625 km/s per megaparsec, which means that two galaxies separated by 1 megaparsec (or 3,26 million light-years) moved away from each other at an apparent speed of 625 km/s, and that two galaxies separated by 10 megaparsecs moved apart at a speed ten times greater.

This unit of measurement, the kilometer per second per megaparsec, shows clearly that the speed of recession depends on the scale. In 1377, in his Book of the Heavens and the World, the scholar Nicole Oresme had noted that, at dawn, one would not notice anything if the world and all living creatures had grown by the same proportion during the night. In Lemaître’s theory, on the contrary, the recession velocity between two points in space grows faster with greater separation, which renders it perceptible.

Lemaître’s article, published in French, passed unnoticed until 1931, when it was finally read by Arthur Eddington, who published an English translation. Unfortunately, this version omits the paragraph in which Lemaître established his law of proportionality, see this article for all the details. Meanwhile, in 1929 the great American astronomer Edwin Hubble had published the experimental results he obtained with his collaborators and described a general law, according to which the speed of recession of a galaxy is proportional to its distance. This law, identical to Lemaître’s, with the same proportionality factor, would from now on carry the name of “Hubble’s law.” It forms the experimental basis for the theory of the expansion of the Universe, of which the big bang models are the fruit.

By a curious historical irony, Lemaître would fall victim to two injustices. Not only does the law of expansion not carry his name, even though he was the first to discover it, but he is also not generally credited with the invention of the theory of the big bang: it is Hubble, once again, even though the latter had always disavowed this interpretation of his measurements!

Moreover, Hubble believed that the proportionality factor between recession speeds and distances was constant over the course of time. This proportionality factor was also baptized “Hubble’s constant.” However, the development of cosmological models coming from general relativity, initiated by Friedmann and Lemaître, showed that this “constant” is not one: it de-pends on time, so much so that it is more correct to call it the “rate of expansion.” Of course, the more distant the galaxy, the greater its apparent speed of recession, but the relation between the two is a little more complicated than a simple law of proportionality. It depends on the curvature of space. The measurement of differences with respect to the strict law of proportionality is in fact one of the experimental methods which allow for evaluation of this curvature. The rate of expansion is therefore a crucial quantity in all current cosmological models, and enormous efforts have been made in order to determine its exact value.

How does one do this? According to the Hubble-Lemaître law, one must measure both galactic redshifts (by using the spectral lines) and, independently, the distances to these galaxies. The first task is done with great precision; the second, on the other hand, remains a fundamental problem.

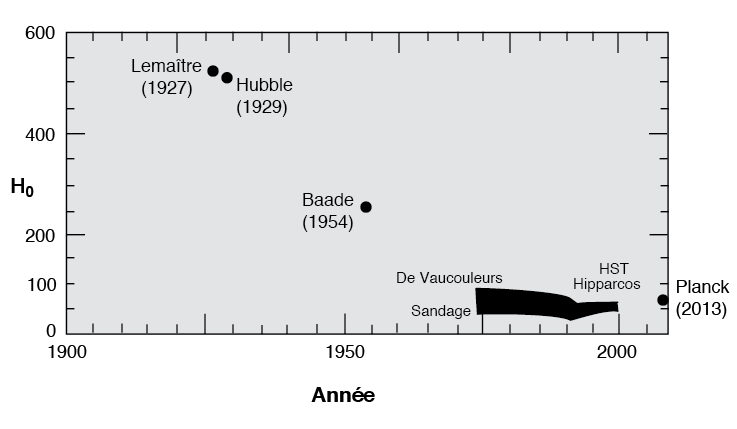
The construction of a trustworthy scale of extragalactic distances has been an immense challenge for astronomers since the 1920s. For a long time, there were two opposing camps, one arguing in favor of a lower value for the rate of expansion, the other in favor of a higher value, with the two values differing by a factor of two!

In reality, each of the two camps evaluated the distances in different ways. Today, the situation has improved considerably thanks to a better understanding of the sources studied and new techniques of observation. Last measurements converge towards a value of 71 km/s per megaparsec (almost ten times smaller than that initially estimated by Lemaître and Hubble[1]), within an error of only a few percent.

 

Now go back to the question of the distance scale on which the expansion of space effectively acts. The solar system, for example: does it grow under the effect of cosmic expansion? The answer is NO. The rate of expansion is imperceptible on such small distances. And the entire galaxy? NO again. Just like any other individual galaxy, it is far too connected by its own gravity for its stars to be dispersed by the expansion. And nearby galaxies? Still NO! United in the middle of groups or clusters, galaxies experience their own peculiar velocities in every random direction. In fact, the spectral lines of the Andromeda galaxy, situated 2 million light-years away, are shifted towards the blue and not the red, indicating a speed of approach of 300 km/s. This is not the effect of a contraction of the spatial fabric between it and us, but simply a movement of free fall, due to gravity! Some other galaxies exhibit a shift towards the blue; but they all belong to our little local Group, including about twenty members, each agitated with its own speed around the center of gravity of the group.

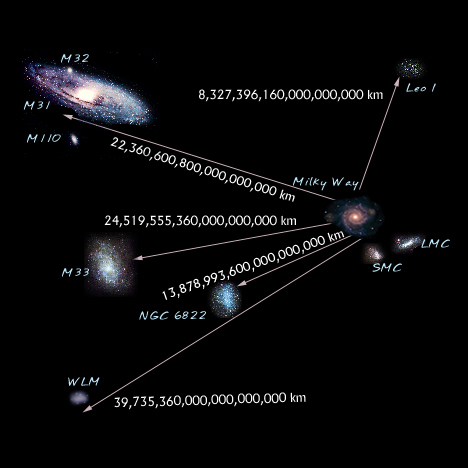
In fact, the distance scale beyond which the expansion acts can be read directly from the value of the rate of expansion rate : 65 km/s for 3 million lightyears, or 650 km/s for 30 million light-years, etc. As long as local speeds are greater than these values, they erase the tendency towards expansion. In all of the clusters, the galaxies have peculiar velocities of a few hundred kilometers per second around the center of gravity of the cluster. The clusters themselves can participate in currents of matter at a large scale, under the gravitational influence of gigantic structures like the superclusters and attractors. Here again, their peculiar velocities are several hundred kilometers per second. The expansion of space therefore only acts definitively above local motions starting from a distance of around 100 million light-years, corresponding to a recession speed of 2,000 km/s. One hundred million light-years is the approximate size of our local Supercluster. It is only beyond this limit that the realm of relativistic cosmology really opens up…

[1] The fact that it has decreased by a factor of ten in the space of 65 years does not reflect its real dependence as a function of cosmic time, but rather the enormous errors in human measurements!