Until now I published as an author 30 books in my native language (French), including 14 science essays, 7 historical novels and 9 poetry collections (for the interested reader, visit my French blog here.
Although my various books have been translated in 14 languages (including Chinese, Korean, Bengali…), only 4 of my essays have been translated in English.
The third one was :
Celestial Treasury: From the music of the spheres to the conquest of space.
Translated from French by Joe Laredo
Cambridge University Press, 2001 — ISBN 0 521 80040 4
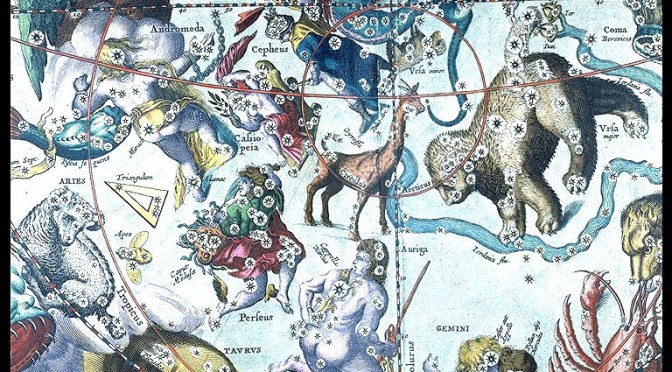
 Throughout history, the mysterious dark skies above us have inspired our imaginations in countless ways, influencing our endeavours in science and philosophy, religion, literature and art. Celestial Treasury is a truly beautiful book showing the richness of astronomical theories and illustrations in Western civilization through the ages, exploring their evolution, and comparing ancient and modern throughout. From Greek verse, mediaeval manuscripts and Victorian poetry to spacecraft photographs and computer-generated star charts, the unprecedented wealth of these portrayals is quite breathtaking.
Throughout history, the mysterious dark skies above us have inspired our imaginations in countless ways, influencing our endeavours in science and philosophy, religion, literature and art. Celestial Treasury is a truly beautiful book showing the richness of astronomical theories and illustrations in Western civilization through the ages, exploring their evolution, and comparing ancient and modern throughout. From Greek verse, mediaeval manuscripts and Victorian poetry to spacecraft photographs and computer-generated star charts, the unprecedented wealth of these portrayals is quite breathtaking.
************************************************************************
PRESS REVIEWS
• Review in Astronomy & Geophysics, 2001 December (Vol.42), 6.32
Big and beautiful
This is such a book as would have the most hardened reviewer reaching for the overworked superlatives. Impressive in size and sumptuous in production, for what is actually quite a reasonable price in present-day terms, it contrives to set forth much of the aesthetic attraction of astronomy both ancient and modern.
Originating as an exhibition catalogue and drawing material from many libraries in Europe, the authors have marshalled a stunning array of historical and modem imagery under the general headings of “The harmony of the world”, “Uranometry”, “Cosmogenesis”, and “Creatures of the sky”. Originally published in French as Figures Du Ciel, a title which implies a much more restricted scope than it actually bas — the English title is far more appropriate — it is here elegantly translated by Joe Laredo. Not the least of its virtues is that as the original edition was jointly published by the Bibliothèque Nationale, the authors have been able to obtain readier access to the treasures of that institution than many other researchers find possible, especially since the move.
Many of the illustrations from conventional astronomical rare books are familiar, though the hand-colouring of different copies makes a fascinating comparison, but others are less so — apart from the unique manuscript sources, the authors have made appropriate use of decorative embossed book covers, illustrations from l9th and 20th century books, especially early science fiction, early space art and even comic books. It can be a trifle disconcerting to find, for example, a modern map of the cosmic microwave background radiation juxtaposed with a l4th century manuscript, but such comparisons can be quite reasonable as long as they are not taken too literally; I feel, though, the series of illustrations comparing the illustrations of the days of creation from the Nuremberg Chronicle with stages in cosmic evolution and the development of life is a little forced. There are one or two isolated nods towards world views outside the main stream leading down from Classical via Arabic to modem western science, but the Hindu Triad and the brief nods towards Chinese, Aztec and Babylonian astronomy seem lonely and isolated and might have been better omitted if there was not room to treat them more fully.
Although the innumerable illustrations are the most prominent feature of the book, the authors’ impeccable credentials as high officials of the CNRS and as successful popularizers of astronomy lend the text authority and style. Occasionally, as used to be said of Sir James Jeans, they get lost in descriptions of immensity and hugeness; but then, in the words of the late Douglas Adams, “Space is big, really big!”. The authors have carefully described the significance of the thought behind the historic images, and the whole book will make a marvellous crib for captions and exhibitions, as well as being ideal fodder for picture researchers. One might pick up small factual disagreements and pedantic quibbles, or take issue with certain aspects of the book production; the truncated and varying sized pages seem to add little but confusion, and I am not clear why the key map from Doppelmaier’s New Celestial Atlas (p108) has been truncated. Lt is also a great shame that a proper index of subjects could not have been added rather than just one of names.
But despite any venial criticism of minutiae, the whole book is a striking demonstration of my own conviction that the most valuable use of historical imagery is to provide an accessible entry point to the subject; such beautiful images, intelligently explained, can engage the interest and commitment of the mathematically challenged in a way that the Schwarzschild Radius or the Chandrasekhar Limit will never do. A book that anybody with the slightest interest in the subject would be delighted to find waiting after the annual visit of the red-coated gentleman with the sub-orbital reindeer!
P D Hingley.
• Review in The Los Angeles Times, March 17, 2002
by Margaret Wertheim
The Sky’s the Limit
Artists and scientists, Robert Oppenheimer wrote, “live always at the ‘edge of mystery’–the boundary of the unknown” and for no group of scientific practitioners is this characterization more apposite than cosmologists, they who dare to envision the universal whole.
Few areas of inquiry bring human minds so constantly into contact with the event horizons of current understanding, so posing the greatest challenges. As a creative response to the ineluctable desire to know how and from whence we arise, cosmological theorizing, for all its claims to truth, is an exercise of the grandest sort in myth-making. That at least is the thesis underlying Marc Lachièze-Rey and Jean-Pierre Luminet’s sumptuous “Celestial Treasury.”
Ostensibly a history of (primarily Western) cosmological thinking, “Celestial Treasury” advances a far more radical agenda. Rather than presenting their subject as a progressive history, onward and upward from pagan darkness to the light of contemporary scientific genius, Luminet and Lachièze-Rey subversively interweave ancient and modern ideas, continually, if gently, alerting the reader to profound resonances between past and present.
For all our superior observational technology, our sophisticated theoretical frameworks and our fiendishly complex mathematics, we are not so far from our forebears as we often like to think.
Consider the ancient Greeks’ idea that everything in the physical world is composed of four basic elements: earth, water, air and fire. Antiquated baloney, you might think, but Luminet and Lachièze-Rey point out that contemporary physics rests on a not-dissimilar premise.
Today the four “elemental” constituents said to be responsible for all phenomena are the four fundamental forces: gravity, the electromagnetic force and the strong and weak nuclear forces (which hold together the nuclei of atoms). These forces, they write, “have an identical function to the elements of the classical world.”
In our drive to know the universe, it is the imagination that engages first, long before the analytical or empiricist spirit kicks in. Johannes Kepler, the great precursor to Isaac Newton and the founding father of modern astrophysics, envisioned the universe as God’s play: As he saw it, the aim of the astronomer was to learn to play God’s game. To do that, the mind must be open to the “facts,” but critically it must also be creatively susceptible. As Albert Einstein once declared: “The gift of fantasy has meant more to me than any talent for abstract, positive thought.”
Throughout history, the creative impulse has been a central engine of cosmological theorizing. Take, for example, the Greek and medieval view that the dance of the planets and stars must be explained by a combination of strictly circular motions. Just as a windup ballerina can be made to perform a complex dance, even though her mechanism consists only of circular gears, so cosmologists for 2,000 years believed the motions of the heavenly bodies could be described by an intricate celestial clockwork.
The apotheosis of this imaginative mechanizing was the dizzyingly elaborate system of the Alexandrian astronomer Claudius Ptolemy. So complex was Ptolemy’s system that in the 13th century Alfonso the Great, seeing the labors of his astronomers, is said to have remarked that had he been present at the Creation he would have given the Lord some hints about simplification. The Ptolemaic conception of the cosmos dominated both Arab and European views of the heavens until the 17th century, when Kepler, Newton and others radically re-envisioned the universe, replacing the cosmic gears with a quasi-infinite network of stellar masses held in place by the force of gravity.
But be not so quick to judge Ptolemy’s vision. Luminet and Lachièze-Rey (an astronomer and astrophysicist, respectively) note that in principle a Ptolemaic-style system could account for the heavenly dance with a high degree of accuracy. In the 19th century, the French mathematician Jean Baptiste Joseph Fourier demonstrated that, in fact, any periodic motion can be described by a combination of circular motions.
Moreover, physics today retains a love affair with the circle. Current favorite contender for a unified theory of the four forces is string theory, which holds that all particles can be understood as the various vibrational states of microscopic circular loops, or “strings.”
Throughout history, cosmological ideas have refracted again and again through our mental prisms, metamorphosing into new variations on old themes. One of the great joys of this book is seeing the ways in which certain tropes keep returning, as if they hold some peculiar power of enchantment over the human mind.
Perhaps my favorite example is the continually recurring fascination with the Platonic solids: a unique set of five forms whose crystalline symmetry has held artists and astronomers, mystics and mathematicians in thrall for thousands of years. As with the cube, whose faces are all squares, the Platonic solids are perfectly regular polyhedra, having all their faces the same. There are just five such forms possible: along with the cube (which has six sides), are the tetrahedron (four sides), the octahedron (eight sides), the icosahedron (20 sides) and the dodecahedron (12 sides). Since their discovery, these five forms have been imbued with almost mystical power.
Plato paired the first four with the four basic elements: Earth was paired with the cube, water with the icosahedron and so on. The fifth, the dodecahedron, he equated with the supposed fifth element, or quintessence, the mysterious substance of which the celestial bodies were said to be composed.
In the 17th century, Kepler thought he had found in these five forms the secret of the planets’ arrangement in the solar system. He turned out to be wrong, but, bizarrely, the idea of a polyhedral arrangement to the cosmos has resurfaced within the framework of general relativity, which allows for some truly extraordinary topologies, including ones in which space takes on a pseudo-crystalline structure.
One such arrangement is an infinite lattice of dodecahedrons. “Celestial Treasury” includes an exquisite computer image of this enigmatic spatial structure from the Geometry Center at the University of Minnesota.
In making their case for cosmological resonances through the ages, Luminet and Lachièze-Rey critically rely not just on words but also on pictures. The uniqueness of this book lies in its juxtaposition of historical images with those generated by contemporary astrophysics, such as the contrasting of Kepler’s polyhedral model with the Minnesota computer model.
Likewise, illustrations from medieval manuscripts of the six days of biblical creation sit side by side with computer simulations of black holes and the origins of space time; Renaissance visions of stellar vortexes are paired with photographs of spiral galaxies taken by the Hubble Space Telescope.
Replete with extended foldouts and delicately detailed inserts, “Celestial Treasury” is a stunningly beautiful survey of the science, mythology and iconography of the cosmos through the ages. This is the most gorgeous coffee-table cosmology book in years.
Such lavish production bespeaks its origins: The book is an offshoot of a 1998 exhibition entitled “Figures du Ciel” at the Bibliothèque Nationale de France, and it is from that library’s extensive collection that most of the older images are taken.
As with two recently ended and superb exhibitions in our own city–“Treasures of the Great Libraries of Los Angeles” at the UCLA Hammer Museum and “Devices of Wonder” at the Getty–“Celestial Treasury” demonstrates that science can be an engine not only of knowledge but also of aesthetic inspiration. Beneath the radar of pedagogical impulse, science, like art, stirs our imaginations.
Margaret Wertheim is the author of “The Pearly Gates of Cyberspace: A History of Space From Dante to the Internet.”
************************************************************************
EXCERPT FROM CHAP. 1 “THE HARMONY OF THE WORLD”
The Fabric of the World
Any macroscopic conception of the universe is also a conception of its microscopic structure. The question whether matter can be broken down indefinitely is an ancient one. The Greeks in particular put forward ideas as to its composition: in keeping with their belief in a rational, unified and harmonious universe, not only did there have to be a limited number of fundamental elements, but there must also be laws governing their combination and transformation.
The Milesian philosophers showed their preoccupation with coherence and their aspiration towards universality by asserting the predominance of one element over all others in the universe: for Thales it was water, for Anaximenes air, for Heraclitus fire and for Anaximander apeiron — a forerunner of Aristotle’s ether.
The search for a single primordial element is a recurring feature of natural philosophy. In the fifth century bc Parmenides of Elea and his pupil Zeno hypothesised that the universe consisted of a single substance, “Oneness”, which was motionless and of infinite mass, enveloping all things without any space between them. In the 12th century ad the English prelate Robert Grosseteste, who is widely considered to be one of the founding fathers of empirical science, regarded light as the most elementary substance and the primary constituent of the world. This idea, which was in keeping with Christian doctrine (God created light, from which everything else followed), is also close to modern thinking, especially that of physicists who are seeking a unified theory of matter and the interaction between bodies.
In the mid-fifth century bc the philosopher and soothsayer Empedocles (who claimed divine ancestry) offered a synthesis of his predecessors’ ideas: he proposed that the primordial material was a combination of the four eternal and incorruptible elements, earth, water, air and fire. In his Origin of the Elements he describes how these elements temporarily combine to form the various sublunary bodies: “Birth is not the beginning of life, and death is not the end; the components of our bodies are merely assembled and disassembled. Birth and death are but the names given to this process by man.”
Elements and Polyhedra
The four element system was adopted by Plato, whose Timaeus describes the sublunary world as subject to a series of transformations – birth-corruption-death – of the four elements brought into being by the Creator. Plato then develops his ideas on representing the cosmos in geometric terms. This sublunary world is far less harmonious than the superlunary world; consequently each of the four elements comprising it must be represented by a shape somewhat less symmetrical, less pleasing and perfect than a sphere, i.e. one of five “Platonic solids”, nowadays known as regular polyhedra. Earth is shown by a cube, water by an icosahedron, air by an octahedron and fire by a tetrahedron (or pyramid). Plato thought carefully about the relationship between each element and its representative shape: for example, a cube is the most difficult shape to move, so it is associated with earth, the heaviest element; an icosahedron has more sides than any other Platonic solid (five triangles meet at each point), giving it a virtually round, fluid structure which is most clearly associated with water; and so on.
Being corruptible, these four elements cannot exist in the sky. There is, however, a fifth regular polyhedron, the dodecahedron, vhich consists of 12 pentagons and is therefore the three-limensional equivalent of the pentagon, considered to be a “magic” nape. To Plato each perfect solid represented the essence of its :orresponding element so, when his contemporary Theaetetus pointed out to him that the dodecahedron was the fifth regular polyhedron (there are only five), Plato postulated a fifth essence in prder to unify his geometric model of the world. In the Middle Ages pis fifth essence was to be known as “quintessence”, but in ppinomis, a work published after Timaeus and generally attributed to Plato, it is called aither, meaning “eternal flux”. Of the five jerfect solids the dodecahedron is the nearest to a sphere, the symbol of celestial perfection.
In general Aristotle appropriated the Platonic model of the :osmos, but he did not adopt the idea of a correspondence between he elements and the regular solids. His main concern was to iistinguish between two worlds, between two kinds of activity. The mperfect sublunary world was governed by “lower activity”: everything is composed of corruptible matter and tends to revert to he natural state of its predominant element. For example, if a stone s dropped, it naturally falls towards the centre of the earth, since :arth is its predominant element. Fire, on the other hand, rises into he air. All natural movement is directed either upwards or iownwards, either towards or away from the centre of the earth [then considered to be at the centre of the cosmos). As for the four dements, they are distinguished by their basic characteristics: earth s cold and dry, air is warm and humid, etc. If these characteristics ire changed, one element can be transformed into another. In the iublunary world such transformations are continually taking place, .vhich accounts for its constantly changing nature.
The Aristotelian picture is completed by the “upper activity” of he superlunary world of planets and stars, whose “physicality”, mquestionably real and tangible as it is, consists of the fifth element, quintessence.
Plato’s polyhedra, which Kepler himself used to account for the orbital motion of the planets, were central to the way the world was represented in succeeding centuries. In the Renaissance, artists such as Piero della Francesca and Paolo Ucello were fascinated by them. phis Divine Proportion of 1509, which was illustrated by Leonardo la Vinci, the mathematician and theologian Luca Pacioli used them 😮 define his laws of just proportion, applicable to music, architecture, calligraphy and other arts.
The Nature of the Ether
After Aristotle the nature of the fifth element changed repeatedly and was the subject of constant debate. In the fourth century BC one of his successors, Theophrastus, rejected the idea of a fifth element altogether, likening the sky to a sort of “ethereal fire” (Manilius, Astronomicon Poeticon). Three hundred years later Xenarchus also dismissed Aristotle’s ether and argued that there could not be only two basic geometric shapes (a straight line and a circle). It was not until the 16th century that the ether was redeemed. Descartes mentions a “subtle substance” filling all space and accounting for his “vortices” and consequently all cosmic interaction. The 17th century Dutch physicist Christiaan Huygens applied the idea to light, which he regarded as a disturbance of an omnipresent “luminiferous ether”. For Newton light was composed of particles and independent of Huygens’ undulatory theory. On the other hand he postulated a kind of “gravitational ether”, a substance occupying all space and capable of transmitting the force of gravity. The idea of light being composed of waves was revived in the 19th century, when it was thought to be transmitted by vibrations in the ether. According to James Clerk Maxwell, electromagnetic waves were also propagated by the ether.
The exact nature of the ether has been the subject of repeated controversy. Does it have physical properties? How does it relate to space, to quantum fields, to a vacuum? Does it move relative to the earth? This last question was the subject of a series of experiments (the most famous being the Michelson-Morley experiment in 1887) and hypotheses leading to the theories of relativity which revolutionised man’s concept of the ether. According to the general theory of relativity, it is the distortion (or curvature) of space-time that conveys gravitational interaction and ripples of space-time that convey energy. The curvature of space-time has exactly the same characteristics as Newton’s “gravitational ether”: it is simultaneously physical and geometric. The other great theory of modern physics, quantum physics (more precisely quantum field theory), presents an alternative view of the ether as the “quantum vacuum”, which has energy and fluctuations but whose nature is still unclear. The concepts of “gravitational ether”and quantum vacuum are central to their respective disciplines, yet the two are irreconcilable. This is the impasse facing modern physics. Its resolution may yet come from further investigation into the nature of the ether, the vacuum, space..
Atoms
The extraordinary popularity of Aristotle’s system of elements meant that the alternative view of matter being composed of atoms, although at least as logical and persuasive, did not develop until the 17th century.
An atomistic structure was proposed as early as the fifth century bc, a generation before Socrates, by Leucippus of Miletus (or Elea) and his disciple Democritus of Abdera, whose work is best known through Aristotle’s Metaphysics. While Leucippus is usually credited with few books, including The Great World System, Democritus was a prolific author known to have written at least 52 books, although some of them are quite short. His Concise World System is a continuation of Leucippus’ work.
Leucippus and Democritus were reacting against the theories of Parmenides and Zeno of Elea, rejecting their idea of an infinite, static, all-embracing substance. They argued that our senses detect movement and that consequently there must be empty space. Their conclusion was a bipartite structure: “The first principles of the universe are atoms and empty space; everything is merely thought to exist.” The four elements of Empedocles have no place in their theory; nor does any such thing as quintessence. Everything is composed of atoms (from the Greek atomos, meaning “indivisible”), which cannot be further broken down because they contain no empty space (for things to be broken apart there must be space between them) and are incredibly compact and heavy. Atoms are also eternal; in other words they have always existed and they cannot change or die. In his treatise De Generatione et Corruptione Aristotle records Democritus’ and Leucippus’ view that these “indivisible bodies” are “are infinite both in number and in the forms which they take, while the compounds differ from one another in their constituents and the position and arrangement of these.”31 The less space there is in a material, the denser it is. Fire, for example, is simply matter whose atoms are widely separated: being of low density it escapes the material that produces it. Celestial bodies are made from the least dense material.
Atomistic theory, which was pursued by Epicurus and Lucretius, was completely overshadowed by Aristotelianism but revived in the 17th century, particularly by the French philosopher Pierre Gassendi, and has become the basis of modern physics, even though our ideas about atoms are quite different from those of the ancient Greek philosophers.
The atom of contemporary science, far from being a compact mass, consists mostly of empty space in which tiny particles revolve around an extremely dense nucleus. The ancient atom in fact corresponds more closely to our idea of an elementary particle. These particles, which constitute the fundamental “building blocks” of every known chemical element, are indivisible masses surrounded by space. The fact that they exist throughout the universe has been proved by spectral analysis of the most distant stars and galaxies.
Harmony and Particles
The regular solids used by Plato and Kepler to represent the elements and the planetary orbits are absolutely symmetrical. To create a concrete model of the planets’ orbits, which were puzzling astronomers, Kepler used geometric shapes that he knew were symmetrical, thereby expressing his vision of cosmic harmony. Today physicists are faced with similar problems: how should they classify the numerous particles which experiments suggest are elementary or fundamental? How should they interpret the similarities and differences between them? How should they analyse their actions and reactions? Like Kepler they have looked to their stock of tools for expressing harmonious or symmetrical relationships for an answer. This they have found in the mathematical theory of groups, which permits the classification of geometric symmetries.
According to modern group theory to each regular polyhedron corresponds a “polyhedral group” (all possible displacements it can be subjected to without changing shape). To a sphere corresponds a higher dimensional group, but even this is just a particular case of a general class of transformations, the “symmetry groups”. In particle physics certain particles are associated with other particles or families of particles, forming “gauge theories”. Gauge theories allow the behaviour of particles – especially the way they interact -to be precisely described. The result is a “harmonious” classification of particles and their interactions, e.g. U(l), SU(2), SU(3).
These theories are undoubtedly more successful than Kepler’s geometric explanation of planetary orbits; yet no physicist would pretend to understand any better than Kepler did where such harmony comes from. He at least was able to interpret it as the will of God!
A Polyhedral Universe
Regular polyhedra appear again in relation to the structure of matter. Although Kepler was forced to abandon them in favour of ellipses as a method of describing the structure of the solar system, he retained his fascination for these almost perfect shapes. In looking at the group of semi-regular polyhedra (rhomboids, prisms, etc.) which incorporates the group of regular solids but also includes non-convex shapes, Kepler discovered “stellation”. In his De Niva Sexangula (The Six-Cornered Snowflake) of 1610 he used five- and six-pointed star shapes to represent the structure of snow crystals, thereby laying the foundation of modern crystallography. In crystallography, polyhedral symmetry reigns supreme. In the 18th century alchemy gave way to the less far-reaching but more rational science of chemistry, which is concerned with the geometric structure of molecules and crystals, underlying that of matter itself. Many molecules have extraordinary structures. The numerous possible arrangements of carbon atoms and other similar atoms, which are rich in symmetry, are often types of polyhedron. An entire branch of organic chemistry, which plays such an important part in modern chemistry, is based on the benzene molecule (C6H ), a beautiful hexagonal shape. Not long ago chemists discovered an even more remarkable molecule: fullerene (C60), which consists of a football-shaped polyhedron surrounded by sixty carbon atoms. First simulated in 1985 this molecule has already generated a new branch of applied chemistry and the number of possible applications for fullerene is still rising. It has recently been detected – as the ion C6+0 – in outer space, where it absorbs light from distant stars, and is the largest molecule (more precisely the largest chemical complex) known to exist in space. It is estimated that such molecules account for some two per cent of all the carbon in the universe.
Polyhedra even have an unexpected relevance to modern cosmology, which is investigating the possibility that space itself is in some way polyhedral and that the cosmos as a whole has a crystallographic structure. According to the general theory of relativity, space has a geometric structure characterised by curvature and topology. This idea fascinated several of the founders of 20th century cosmology such as De Sitter, Friedmann and Lemaitre; it then rather lost favour before regaining popularity in recent years. In “topologically multi-connected” models, which can initially seem confusing, space is represented by a “fundamental polyhedron”. The simplest of these models use cubes or parallelepipeds (shapes consisting of six parallelograms) to create a “toroidal” space, but there is an almost infinite number of variations. The common feature of these fundamental polyhedra is that they are symmetrical, so that one face can be related to another: the corresponding points on each face are therefore “linked” in such a way that physical space is the result of a complex “folding” process. The fundamental geometric symmetry of the universe is matched by the symmetry of the polyhedron.
From the point of view of the celestial observer, these “folded” models introduce a radically new perspective. The usual interpretation of the sky is that it consists of a straightforward projection of the space, which is vast if not infinite: each point of light that we can see corresponds to a specific star, galaxy or other celestial body – the further away the fainter. This is not at all the case with a multi-connected model, according to which each actual celestial body is represented by a whole series of “ghosts” so that what we see in the sky is not the universe as it really is, but several different images of the universe, from different angles and distances, superimposed upon one another!
Symmetry in Modern Physics
Symmetry is one of the most fundamental concepts in geometry, whose principal concern is to find “pure” shapes – the equivalent of the physicist’s search for fundamental elements. One of the simplest symmetrical shapes is the sphere, which is symmetrical with respect to any straight line passing through its centre. Others are the regular polyhedra (the Platonic solids), which are symmetrical with respect to a finite number of lines passing through their centre.
Symmetry is so prevalent in nature – from the human body to atoms and crystals – that it is difficult to imagine it not being central to our understanding of the world and its creation. Although symmetry was studied by the French mathematician Evariste Galois in the early 1830s and by the German Emmy Noether around 1916, its importance was not fully understood until the development of group theory later in the 20th century. Symmetry is also omnipresent in the arts. The (subjective) notion of beauty is, however, often associated with a slight asymmetry. The most beautiful faces are not exactly symmetrical; the best architects mix the symmetrical with the unexpected. Similarly physicists study symmetry breakdowns and show how these are as fundamental to nature as symmetry itself.
In recent times physics has become increasingly concerned with geometry, emphasising the “Platonic” nature of modern science. A striking example is quantum theory and in particular the theory of elementary particles; in attempting to describe the structure of atoms, in other words the invisible universe, particle physicists have resorted to abstractions based on geometric concepts. Just as Pythagoras himself would have approved of the quantum numbers representing the various levels of placement of electrons around an atomic nucleus, so Plato would have been delighted by the shape of the mathematical wave functions describing the hydrogen atom.