Sequel of the preceding post The Warped Science of Interstellar (1/6)

One year ago, in november  2014, the Hollywood blockbuster and science-fiction movie Interstellar was released on screens and  much mediatic excitation arose about it.

This is the second of a series of 6 posts devoted to the analysis of some of the scientific aspects of the film, adapted from a paper I published last spring in Inference : International Review of Science.

THE FAST-SPINNING BLACK HOLE « GARGANTUA »

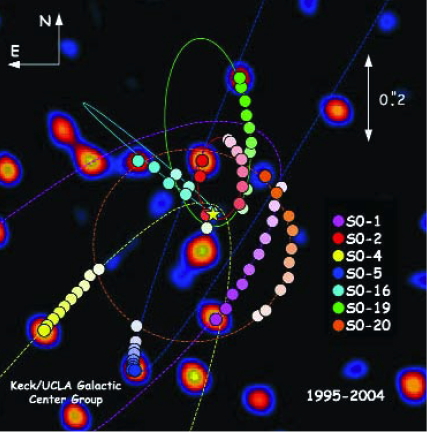
Once on the other side of the wormhole, the spaceship and its crew emerge into a three-planets system orbiting around a supermassive black hole called Gargantua. Supermassive black holes, with masses going from one million to several billion solar masses, are suspected to lie in the centers of most of the galaxies. Our Milky Way probably harbors such an object, Sagittarius A*, whose mass is (indirectly) measured as 4 million solar masses (for a review, see Melia[i]). According to Thorne, Gargantua would be rather similar to the still more massive black hole suspected to be located at the center of the Andromeda galaxy, adding up 100 million solar masses[ii]. Its size being roughly proportional to its mass, the radius of such a giant would encompass the Earth’s orbit around the Sun.

Such enormous black holes are not a science-fiction exaggeration, since we have the observational clues of the existence of « Behemoth » black holes in faraway galaxies. The biggest one yet detected lies in the galaxy NGC 1277, located at 250 million light-years ; its mass could be as large as 17 billion solar masses, and its size would encompass the orbit of Neptune[iii].

Another – and very important – characteristic of Gargantua is that it is a fast-spinning black hole. All the objects in the universe – except the universe itself – rotate. Thus a natural black hole must do so, and be described by the Kerr geometry. The latter now depends on two parameters : the black hole mass M and its angular momentum J. An important difference with usual stars, which are in differential rotation, is that the Kerr black holes are rotating with perfect rigidity : all the points on their surface (the event horizon) move with the same angular velocity. There is however a critical angular momentum J_max above which the event horizon would « break up » : this limit corresponds to the horizon having a spin velocity equal to the speed of light. For such a black hole, called « extremal », the gravitational field at the event horizon would cancel, because the inward pull of gravity would be compensated by the huge repulsive centrifugal forces.

It is quite possible that most of the black holes formed in the real universe have an angular momentum rather close to this critical limit. For instance, a typical stellar black hole of 3 solar masses, believed to be the engine of many binary X-ray sources, must rotate at almost 5000 revolutions per second.

For reasons that will be explained later, the black hole Gargantua shown in Interstellar was assumed to have an angular momentum as close as 10^-10 to the critical value J_max. If theoretically possible, this configuration is physically quite unrealistic, because the more a black hole rotates fast, the more the material orbiting in the same direction is hard to capture, due to the centrifugal forces, while the matter orbiting in the opposite sense is easily sucked into the hole, where it slows the spin. As a consequence, a too-fast spinning black hole would have the tendency to slow down to an equilibrium velocity smaller than that of Gargantua (general relativistic calculations say that black holes spin no faster than about 0.998 J_max).

However, the advantage of a very fast-spinning black hole is that it permits to have planets orbiting extremely close to the event horizon without being swallowed. And this is a key point of the movie, for it also allows for a huge time dilation, see below. For a Schwarzschild black hole (i.e. with angular momentum J=0), the innermost stable circular orbit, inside which any object would spiral and crash into the black hole, is located at 3 times the black hole radius. For a 100 million solar masses black hole, this gives a minimum distance of 900 million kilometers (a little bit more than Jupiter’s distance to the Sun). But for a Kerr black hole spinning very close to the critical limit J_max, the innermost stable circular orbit can be as close as the event horizon itself : 100 million kilometers only. It is the reason why, in Interstellar, the closest planet (called Miller) can orbit safely very close to the event horizon without being swallowed.

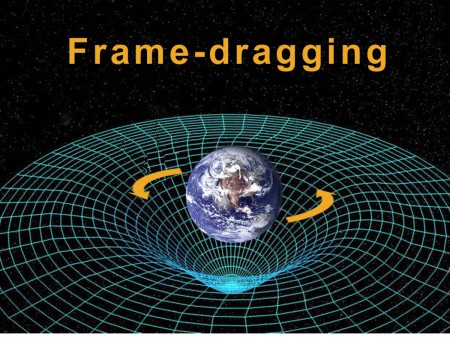
Another noticeable point is that a Kerr black hole is not a spinning top revolving in a fixed exterior space : as it rotates, it drags the entire fabric of space-time along with it. As a consequence, Miller’s planet must orbit at a velocity close to the speed of light.

ILLUMINATED PLANETS ?

All right for the gravitational safety of the three-planets system around Gargantua. But where do these planets get heat and light? In principle a star is needed for that, but there is no star around. Heat cannot come from the black hole itself in the form of the Hawking radiation or the recently advanced « firewall » phenomenon[iv] : these effects being purely quantum in nature, they could be noticeable for microscopic black holes, but are completely negligible for astrophysical ones. Can light and heat come from the gaseous ring that orbits around Gargantua, called an accretion disk ?

Such an explanation is not very consistent with the rest of the story, because later in the movie Cooper inevitably has to go inside the black hole, and he does not get fried. The theory of accretion disks around black holes was actually developed decades ago (for a review, see Abramowicz[v]) and are in agreement with recent astrophysical measurements using gravitational lensing[vi]. Because of the incredible forces involved, accretion disks are extremely hot, like millions of degrees hot. They are so brilliant that they can be seen millions of light-years away, and blast out enough radiation to completely destroy any normal material. Thus the astronauts would have been fried as soon as they emerged from the wormhole. Happily for the continuation of the story, it was not the case. So, how the planets can be habitable despite no nearby source of warmth ?

In his popular book as well as in various interviews, Thorne claimed that the light could come from a very « anemic » accretion disk, that has cooled down to the temperature of the Sun basically (5500 °C). Here « anemic » means that the disk has not been fed by new gas (coming for instance from a tidally disrupted star, see below) in the last million years, and that the accretion rate onto the black hole, a critical parameter on which depends the disk luminosity, is extremely low. In that sense, such a quiescent accretion disk could be relatively safe for humans. But I doubt that it could provide enough light and heat to the planet, like the Sun to us, just because an anemic accretion disk would also be optically thin (i.e. transparent), while the Sun’s photosphere is optically thick (i.e. opaque).

Go to the next post The Warped Science of Interstellar (3/6)

REFERENCES

[i] F. Melia : The Galactic Supermassive Black Hole. Princeton: Princeton University Press (2007).

[ii] R. Bender al. : HST STIS Spectroscopy of the Triple Nucleus of M31: Two Nested Disks in Keplerian Rotation around a Supermassive Black Hole. Astrophysical Journal 631 (1): 280–300 (2005).

[iii] R. van den Bosch et al. : An over-massive black hole in the compact lenticular galaxy NGC 1277, Nature 491, 729 (2012).

[iv] A. Almheiri, D. Marolf, J. Polchinski and J. Sully : Black holes: complementarity or firewalls? Journal of High Energy Physics 2013 (2).

[v] M. A. Abramowicz and P. Chris Fragile : Foundations of Black Hole Accretion Disk Theory, Living Rev. Relativity, 16 (2013)

[vi] S. Poindexter et al. : The Spatial Structure of An Accretion Disk, The Astrophysical Journal, 673 (1): 34 (2008).